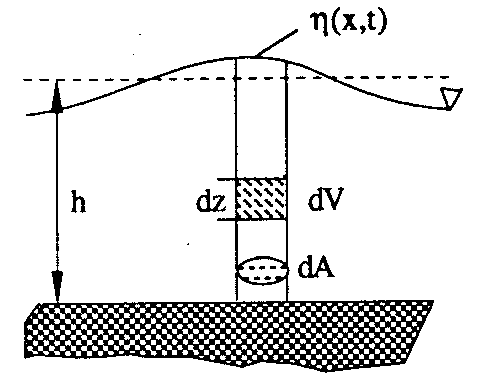
The potential energy contained in a column of water with cross section dA as shown on the graph is
![]()
9 ENERGY AND GROUP VELOCITY
When we look at waves breaking on a shore, it is obvious that the waves bring with them a lot of energy. The energy content in an infinite plane wave is obviously infinite, so we are more interested in finding the energy per unit area of the surface.
|
|
The potential energy contained in a column of water with cross section dA as shown on the graph is
|
Since only the excess potential energy is of interest, we subtract the part corresponding to the mean surface and obtain the potential energy per unit area:
![]() .
.
Instead of using the instantaneous value of h
, it is more common to use the average of ![]() on the right hand side. The average of
on the right hand side. The average of ![]() for a sinusoidal wave with amplitude a is
for a sinusoidal wave with amplitude a is ![]() (We shall return to this in Part B). For a plane wave with amplitude a,
the average potential energy per unit area is therefore
(We shall return to this in Part B). For a plane wave with amplitude a,
the average potential energy per unit area is therefore
![]()
where the brackets are used for indicating the average value.
The kinetic energy is derived similarly by observing that
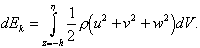
If we (for simplicity) consider deep water and a plane wave ![]() , we obtain
, we obtain
![]()
and therefore
![]()
The average kinetic and the potential energies are thus equal (Show that the error introduced by replacing h by 0 is negligible).
The energy is carried along with the waves, but somewhat surprisingly, the energy is not travelling with the phase velocity of the wave. As a matter of fact, in deep water, the transport velocity of the energy is only half the phase velocity!
We shall not give a rigorous proof of this, but follow a more intuitive argument. Consider first the superposition of two plane waves in the same direction, but with slightly different frequencies and wavenumbers:
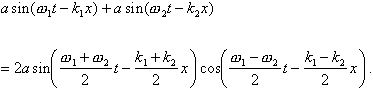
The result is a product of two travelling waves. The first wave has frequency about the same frequency and wavenumbers as the two original waves, whereas the second wave has frequency ![]() and wavenumber
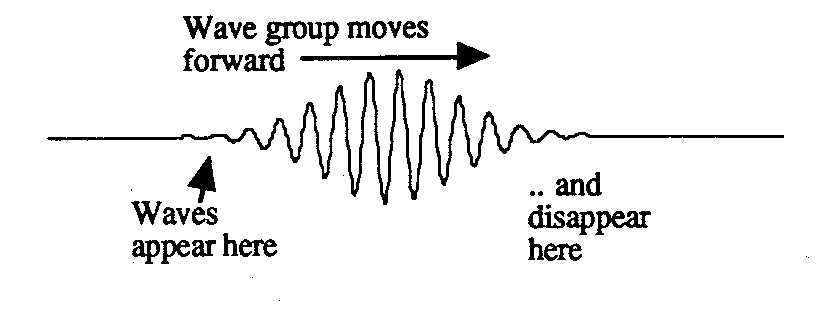
and wavenumber ![]() . At a given instant of time, the result will look as shown on the figure.
. At a given instant of time, the result will look as shown on the figure.
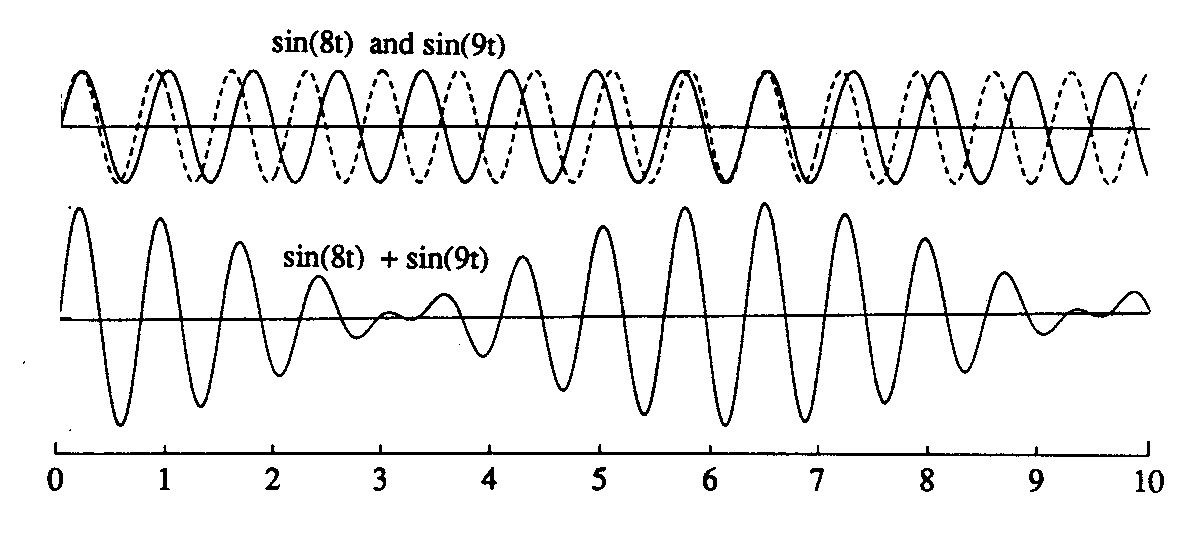
The result consists of "groups of waves" moving with the phase velocity of the "cosine" wave:
![]() .
.
If we are sitting in a boat in one of the minima for the amplitude (called "knots"), and moving with a velocity equal to ![]() , we would not feel any waves at all! And energy will pass us in either direction. From this we conclude that the energy is moving with the same speed as we are, namely the group velocity, which in the limit amounts to
, we would not feel any waves at all! And energy will pass us in either direction. From this we conclude that the energy is moving with the same speed as we are, namely the group velocity, which in the limit amounts to
![]()
Since we are dealing with water waves fulfilling the dispersion relation, we have
![]()
In deep water (![]() ) we obtain from the expression above, or simply from the corresponding dispersion relation that
) we obtain from the expression above, or simply from the corresponding dispersion relation that
![]()
In deep water, the group velocity is only half the phase velocity. If we watch wave groups, the individual waves are created at the end of the group, and move forwards until they disappear at the front of the group.
In very shallow water, ![]() , and
, and
![]() .
.
In very shallow water, the phase and the group velocities are equal!
Try out: DalrymplesJavaAppletsCoastalEngineering - Superposition of Waves.
For wave power generation, it is the energy coming into the device per time unit rather than the energy content itself which is of interest. In order to derive the available energy, it is convenient to consider an ideal (100%) wave energy absorber placed in front of an incoming plane wave as shown on the figure below. We are interested in the absorbed energy per time unit and length unit of the absorber.
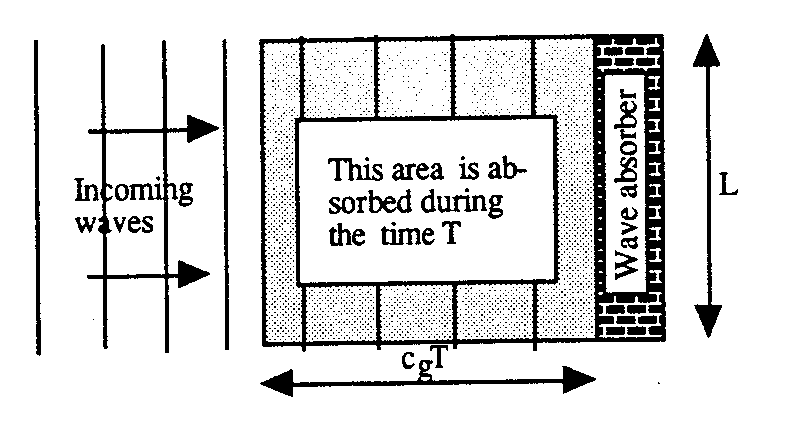
During a time interval T, all energy within the dashed area is absorbed by the wave absorber. The energy within the square is
![]()
The absorbed energy per time unit and per length unit of the absorber is thus
 .
.
In metric units, the unit for J is Watt per meters (W/m).
Consider a typical ocean wave with amplitude a=10m and period T=10s. In deep water,
![]()
Thus,
![]()
This is considerable! Unfortunately, existing wave power devices are far from being "perfect".